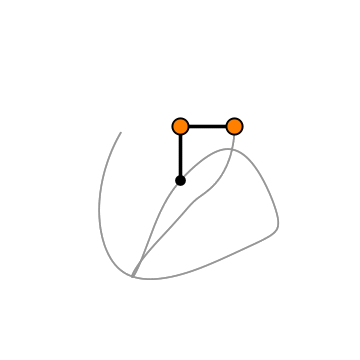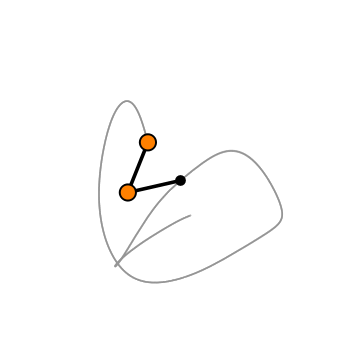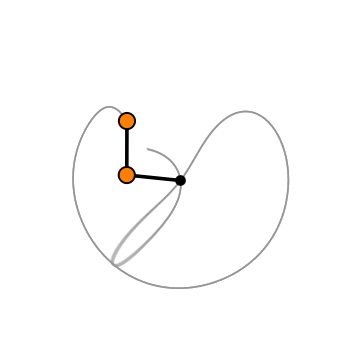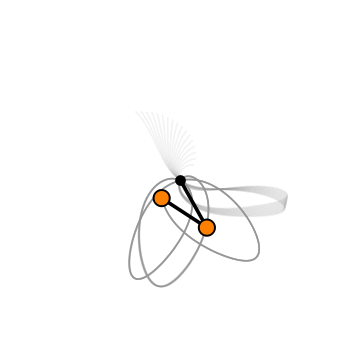
Bestand:Double pendulum predicting dynamics.gif
Naar navigatie springen
Naar zoeken springen
Double_pendulum_predicting_dynamics.gif (360 × 360 pixels, bestandsgrootte: 1,84 MB, MIME-type: image/gif, herhalend, 334 frames, 33 s)
Let op: vanwege technische beperkingen, kunnen miniaturen van GIF-afbeeldingen met een hoge resolutie niet geanimeerd worden weergegeven.
Bestandsgeschiedenis
Klik op een datum/tijd om het bestand te zien zoals het destijds was.
| Datum/tijd | Miniatuur | Afmetingen | Gebruiker | Opmerking | |
|---|---|---|---|---|---|
| huidige versie | 6 jul 2021 09:55 |  | 360 × 360 (1,84 MB) | Berto | Uploaded own work with UploadWizard |
Bestandsgebruik
Dit bestand wordt op de volgende pagina gebruikt: